How does gravitational slingshotting work?

By orbiting the planet (or whatever object) that is generating the gravitational field, the spacecraft actually gains the velocity of the planet.

Here’s a good (simplified) explanation from Wikipedia. There is loss of energy from the planet, but given the relative size of the spacecraft to the planet, it is negligible.

Do I have that correct, @LuckyGuy?

I love this question! The reference above gives a good explanation but I will give a really simple one.
You asked: “Where does the energy come from?” It comes from the heavier object, (planet, moon, sun, asteroid, etc) the spacecraft is swinging around. The planet slows down just a touch while the spacecraft speeds up. It’s a neat trick that permits spacecraft to travel fast and far without carrying so much fuel.

The Laws of Physics are quite orderly and predictable so the entire mission is planned before launch. Incredible!

You can also use slingshotting to slow down a craft. “Look Ma! No brakes!”

@LuckyGuy I am thinking that would be by approaching the orbiting object more in the same direction as the orbit?

Apollo 13.

Here’s an analogy. Two flies smell a dumpster on the other side of the playground. A race ensues.

The first fly gets a head start. He sees a stationary basketball on the ground. He decides to slingshot around its gravitational field. As he dips into the field, he gains velocity. It’s working! But, as expected, the energy needed to escape the ball’s field slows him down again. Damn!

The second fly sees Napolean Dynamite playing tether ball. He makes some quick calculations. He determines that if he approaches with just the right timing, velocity and trajectory, he can dip into the ball’s gravitational field while it is in orbit. High risk, high reward.

He times his arrival perfectly. As he dips into the field, he gains velocity from the orbit while the gravitational field keeps him with the ball. As he escape’s the ball’s gravity, he shoots out across the playground and splatters into the side of the dumpster. Victory!

Here’s another primer on the subject of Gravitational Assist from NASA Jet Propulsion Lab at Caltech.

I appreciate the question and the answers, but I am having trouble understanding why the spacecraft should pick up twice the planet’s speed. It is not really bouncing off the surface of the planet. The analogy that I picture is of someone running along a railroad track and then a train comes along. The person jumps onto the train, still running at the same rate. The person’s speed relative to the ground is the running speed plus the train’s speed, and that would continue to be the speed relative to the ground if the runner jumped off the train.

@LostInParadise I am similarly confused. Special relativity holds that there is no such thing as objective velocity. Velocity can only be expressed relative to other things. If you slingshot around the Sun, where does your extra speed relative to the Sun come from? Does this mean that you can only slingshot in the direction of objects approaching the object from which you’re slingshotting? How does this work when slingshotting from a gravitational mass in orbit around another gravitational mass? For example, when you slingshot around Jupiter, do you gain or lose velocity relative to the Sun?

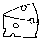
It wouldn’t work to slingshot around the sun because it doesn’t have an independent orbit. If the sun is the tether ball in my analogy, then we must imagine the entire playground rotating with it. From the bug’s perspective, the tether ball would be stationary, like the basketball.

To determine if a spaceship will gain or lose velocity (relative to the sun) when it slingshots around Jupitor, we need to consider the spaceship’s direction of travel relative to Jupitor’s. To increase velocity, the spaceship must exit at an angle closer to Jupitor’s than it was traveling before the encounter.

In this image, the spaceship is purple and Jupitor is black. Trajectories (a), (b) and (d) slow it down while (f), (h) and (i) speed it up.

Here is the article.

@Lawn So slingshotting around the Sun does work if you’re heading for another star relative to galactic centre, right?

@SmashTheState Yes, it would works in those cases. But that would be one loooog mission. It would take thousands of years to reach the other star. Remember you can’t let the craft swing too close to the sun or it would burn up. Swinging close to a planet is fine as long as you stay away from its atmosphere.
Just for fun let’s do a fist order approximation for mission length. Let’s use Voyager as an example using no calculator.. Just use rounding. Voyager has been heading out for nominally 40 years and is nominally 4 light hours away so let’s figure 1 light hour every 10 years . A nearby star is 6 light years. 365 days x 24 hours, call it 400×20 or 8000 hours per light year; times 6 give 48000 light hours to nearby star . Now multiply by 10 years per light hour and you get 480,000 years. Try getting that project funded.

You can swing around the sun at a safe distance to aim the craft for multiple gravitational assist moves around planets. .

@LuckyGuy You can swing around the sun at a safe distance to aim the craft for multiple gravitational assist moves around planets.—Solar Pinball?

I think it depends where we start. The sun’s orbital velocity around galactic center is roughly 500,000 MPH. If we depart from Earth (or anywhere within the Solar System), then we’re already along for the ride (like an oxpecker on a wildebeest). There’s no angular momentum to “steal” from the sun’s orbit because we’re already in it.

If we go back to the NASA train analogy, the sun is the train and the 50 MPH represents its orbital velocity relative to galactic center. The kid with the propeller is Earth, only now he’s flying right along with the train (imagine him orbiting the front of the train as it moves). When he throws his ball (spaceship) at 30 MPH, it bounces off at 30 MPH. He could have just thrown the ball the other direction. There would be nothing to gain from the solar “fry-by” originating within the Solar System. It would be equivalent to (e) in the image I linked above.